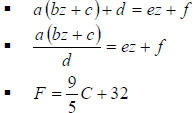MATH 81 MASTERY GOALS
| Topics & Sections of Text | Details |
| Fraction and signed number arithmetic Main idea: Accurately and efficiently compute with fractions and signed numbers mentally or by hand. Relevant sections of text: (1.3, 1.5 – 1.7) Outcomes Assessed: o Students will perform arithmetic of rational numbers (both with and without a calculator). |
The student should be able to: • Explain what an individual fraction or integer means and provide an example of where they’d encounter it. • Add, subtract, multiply, and divide fractions with unlike denominators, then simplify results. o Advanced ability: do the same with mixed numbers • Add, subtract, multiply, and divide one- and two-digit integers. o Advanced ability: do the same with rational numbers, and decimals to the tenths
|
| Interpreting and creating graphs Main idea: Accurately and efficiently interpret and create graphs of data. Relevant sections of text: (3.1, 3.2, 3.3) Outcomes Assessed: o Developing Information and Visual Literacy: The ability to … comprehend, translate between, and reason fluently with multiple representations of the information. o Communicate effectively.
|
The student should be able to: • Interpret information given in a variety of forms: graphs, tables, symbols, and words. • Represent results in, and translate results between different forms. • Evaluate the accuracy and effectiveness of a representation. • Use pie, bar, or line graphs of real data to answer questions about o Values for particular categories/x-coordinates [Equation concept] o Differences between values o Trends * Answers should include correct units o Advanced ability: Answer questions about the categories or x-coordinates at or above (below) a given y-value [Inequality concept] • Create accurate graphs of sets of paired data that effectively support a claim. • Graph a linear equation of the form y = mx + b by plotting points when m and b are integers or simple decimals. o Advanced ability:  Graph ax + by = c . Describe what it means to be a
solution of an equationin two variables. |
| Using and manipulating formulas Main idea: Accurately and efficiently evaluate formulas using substitution and a calculator. Relevant sections of text: (1.4, 1.8, 2.1, 2.6) Outcomes Assessed: o Students will be able to use order of operations correctly to simplify algebraic expressions.
|
The student should be able to: • Evaluate basic science, business, and math formulas involving o at most three variables, o at most three of +, –, ×, ÷, ^, and ( ), and o integer or single-digit decimals. o Advanced ability: do the same with more complex formulas involving exact answers, absolute values, and rational numbers.• Simplify basic abstract expressions like o ax + b + cx + d (x) o a (b + cy)+ d with simple decimal or integer coefficients (at most two are negative). o Advanced ability: simplify more complex expressions like −(ax + b)+ c (d + ex) as well as the previous forms with
rational coefficients. |
| Solving linear equations in one variable Main idea: Accurately and efficiently solve versions of ax + b = c that include only one of the following variants: fractional coefficients, integer coefficients, decimal coefficients, parentheses, variable on both sides. Relevant sections of text: (2.1 – 2.4) Outcomes Assessed: o Students will be able to solve linear equations.
|
The student should be able to: • Understand the different goals for simplifying and solving, and recognize which is possible in a given situation. • Simplify basic equations like o am + b = c o a t + b = c + d t o a = b(c + dw) o P = 2L + 2W , d = rt where coefficients and solutions are integers or simple decimals (at most two are negative). o Advanced ability: Solve equations like
as well as the previous forms with rational
coefficients and |
