Intermediate Algebra

COURSE DESCRIPTION

MATD 0390 INTERMEDIATE ALGEBRA (3-4-0). A course designed to develop the skills and understanding contained in the second year of secondary school algebra. Topics include review of properties of real numbers, functions, algebra of functions, inequalities, polynomials and factoring, rational expressions and equations, radical expressions and equations, quadratic functions and their graphs, solving quadratic equations, and exponential functions. The same course is sometimes offered in a one hour (0190) and two hour (0290) format.

PREREQUISITE

Grade of C or better in Elementary Algebra, MATD 0370, or its equivalent knowledge, or a passing score on the MATD 0390 placement test.

COURSE RATIONALE

This course is designed to prepare students for various college-level science and mathematics courses. After succeeding in this course, students may enroll in a number of courses in science, mathematics and various technical areas. These include General College Physics, General Chemistry, Magnetism and DC Circuits, AC Circuits, Manufacturing Materials and Processes, Math for Business and Economics, and College Algebra.

COMMON COURSE OBJECTIVES

Overall objectives:
 A. Students will feel a sense of accomplishment in their increasing ability to use mathematics to solve problems of interest to them or useful in their chosen fields. Students will attain more positive attitudes based on increasing confidence in their abilities to learn mathematics.
 B. Students will learn to understand material using standard mathematical terminology and notation when presented either verbally or in writing.
 C. Students will improve their skills in describing what they are doing as they solve problems using standard mathematical terminology and notation.

Computational:
 1. Evaluate a function using function notation.
 2. Find the domain of a function.
 3. Perform elementary arithmetic operations with functions.
 4. Perform elementary arithmetic operations with rational expressions that require factoring up to and including the sum or difference of cubes.
 5. Simplify a complex fraction, including one with negative exponents.
 6. Simplify an expression with fractional exponents.
 7. Simplify a radical expression, including rationalizing a monomial or binomial denominator.
 8. Perform elementary arithmetic operations with complex numbers.

Equation and Inequality Solving:
 1. Solve an absolute value equation.
 2. Solve an absolute value inequality of the form |x|<5 or |z|>6.
 3. Solve a rational equation, including one with a quadratic expression in the denominator.
 4. Solve an equation with one radical.
 5. Recognize an extraneous root.

Using Forms and Formulas
 1. Graph a function, such as a simple absolute value or rational function, by completing a table and plotting points.
 2. Solve a quadratic equation with real or non-real solutions.
 3. Find the midpoint and the distance between two points.
 4. Complete a square to rewrite an equation for a circle in standard form and identify its center and radius.
 5. Determine if a formula, correspondence, table or graph represents a function.

Graphing:
 1. Graph a linear inequality on the Cartesian plane.
 2. Graph a system of linear inequalities on the Cartesian plane.
 3. Graph and analyze a linear and quadratic function.
 4. Sketch a quadratic function, written in the form f(x)=a(x-h)^2+k, using transformations.
 5. Graph exponential functions using tables.
 6. Sketch a circle from its standard form.

Applications:
 1. Represent English descriptions of numerical relationships in algebraic form.
 2. Solve application problems including, but not limited to, linear and quadratic models, direct and inverse variation, and those requiring 2x2 systems of linear equations.

COURSE EVALUATION / GRADING SCHEME

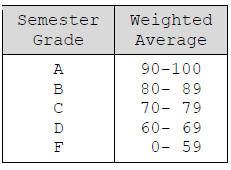
Each student’s semester grade is determined by a weighted average of exam scores, the final exam score, and homework scores. All exams and homework must be completed in pencil.

Exams
There will be four exams and a comprehensive final exam. The exams will each count 15% toward the semester grade. The final exam will count 20% toward the semester grade. Students may not leave the classroom while taking an exam.

Homework
Homework assignments are listed on a separate handout. The homework average will count 20% toward the semester grade. Solution details must be shown in the homework to receive credit. Homework must be completed neatly in pencil on standard size loose-leaf notebook paper.

Homework is due at the beginning of class. One randomly chosen problem from each section will be graded. The lowest three homework grades will be dropped.

MISSED EXAM POLICY

A make-up exam will be given for a student only if the student does not take the exam during the scheduled time in class. Make-up exams are given in the San Marcos Testing Center. Please read the Student Guide for Use of ACC Testing Centers that accompanies this handout.

Each student is permitted to take only one make-up exam during the semester, and make-up exams must be taken on the dates that appear on the assignment handout. Since unforeseen circumstances may prevent arrival to the Testing Center, students are strongly encouraged to take make-up exams on the earliest date possible.

ATTENDANCE POLICY

Attendance is required in this course. Students who miss more than 4 classes may be withdrawn. Student are responsible for the material covered and any assignments that are due for the class period missed. See also the Texas Success Initiative (TSI) Warning below.

TSI WARNING
If you are relying on this course to meet a requirement that you be in mandatory remediation in mathematics this semester*, then:
 i) if you are not "continually in attendance" in this course, you should be withdrawn from the course by your instructor,  

 ii) if you withdraw yourself from this course or are withdrawn by your instructor, you may be withdrawn from all of your other college courses if this is the only TSI-mandated course you are taking.

*If you are unsure whether or not this warning applies to you, see an ACC advisor immediately

CLASS PARTICIPATION EXPECTATIONS

Each student is expected to participate in all course activities.

WITHDRAWAL POLICY

It is the student’s responsibility to initiate all withdrawals in this course. The instructor may withdraw students for excessive absences (4) but makes no commitment to do this for the student. After the withdrawal date, neither the student nor the instructor may initiate a withdrawal. The last day to withdraw from a course this semester is April 27, 2009.

REINSTATEMENT POLICY

Students who withdraw or are withdrawn generally will not be reinstated unless they have completed all course work, projects, and tests necessary to place them at the same level of course completion as the rest of the class. After the last day to withdraw, neither the instructor nor the student may initiate reinstatement into the course.

INCOMPLETE GRADE POLICY

Incomplete grades (I) will be given only in very rare circumstances. Generally, to receive a grade of "I", a student must have taken all examinations, be passing, and after the last date to withdraw, have a personal tragedy occur which prevents course completion.

IN PROGRESS GRADE POLICY

In Progress (IP) grades are rarely given. In order to earn an "IP" grade the student must remain in the course, be making progress in the material, not have excessive absences, and not be meeting the standards set to earn the grade of C or better in the course. Students who are given an IP grade must register and pay tuition for the same course again to receive credit. Students who make a grade of IP should not go on to the next course.

INSTRUCTIONAL METHODOLOGY

This course is taught in the classroom primarily as a lecture/discussion course.

LATE WORK POLICY

No late work will be accepted.

ELECTRONIC DEVICES

Cell phones, iPods, pagers, laptop computers, or any device that may distract from the class should be turned off before entering the classroom and may not be on the desk during class or exams.

COLLEGE POLICY STATEMENTS

Statement on Students with Disabilities
Each ACC campus offers support services for students with documented physical or psychological disabilities. Students with disabilities must request reasonable accommodations through the Office of Students with Disabilities on the campus where they expect to take the majority of their classes. Students are encouraged to do this three weeks before the start of the semester. Students who are requesting accommodation must provide the instructor with a letter of accommodation from the Office of Students with Disabilities (OSD) at the beginning of the semester. Accommodations can only be made after the instructor receives the letter of accommodation from OSD.

Statement on Scholastic Dishonesty
Acts prohibited by the college for which discipline may be administered include scholastic dishonesty, including but not limited to, cheating on an exam or quiz, plagiarizing, and unauthorized collaboration with another in preparing outside work. Academic work submitted by students shall be the result of their thought, work, research or self-expression. Academic work is defined as, but not limited to, tests, quizzes, whether taken electronically or on paper; projects, either individual or group; classroom presentations; and homework.

Statement on Scholastic Dishonesty Penalty
Students who violate the rules concerning scholastic dishonesty will be assessed an academic penalty that the instructor determines is in keeping with the seriousness of the offense. This academic penalty may range from a grade penalty on the particular assignment to an overall grade penalty in the course, including possibly an F in the course.

Statement on Academic Freedom
Institutions of higher education are conducted for the common good. The common good depends upon a search for truth and upon free expression. In this course the professor and students shall strive to protect free inquiry and the open exchange of facts, ideas, and opinions. Students are free to take exception to views offered in this course and to reserve judgment about debatable issues. Grades will not be affected by personal views. With this freedom comes the responsibility of civility and a respect for a diversity of ideas and opinions. This means that students must take turns speaking, listen to others speak without interruption, and refrain from name-calling or other personal attacks.

Statement on Student Discipline
Classroom behavior should support and enhance learning. Behavior that disrupts the learning process will be dealt with appropriately, which may include having the student leave class for the rest of that day. In serious cases, disruptive behavior may lead to a student being withdrawn from the class.